A11 – Camera Calibration

In this activity we try to calibrate our cameras. We do this by taking an image of a 3D calibration checkerboard. Then we assign a an origin and a right-handed coordinate system in the grid and measure around 20 to 25 corners of the squares of the checkerboard pattern. Each corner would have a coordinate [Xw, Yw , Zw]. From the image of the grid we get the coordinate [Yi, Zi] of each corners of the squares that we chose. These are the data that I have gathered:

Xw	Yw	Zw	Yi	Zi
0	0	0	100	36
1	0	2	90	72
1	0	4	90	112
0	1	5	117	131
0	3	7	154	168
0	4	4	171	106
0	3	2	152	69
0	6	1	205	44
0	5	8	191	185
4	0	7	57	164
5	0	4	46	100
4	0	1	58	42
2	0	9	80	208
7	0	2	23	52
6	0	8	35	181
0	7	6	228	142
0	4	10	173	227
5	0	10	47	225
0	2	9	137	208
0	5	2	188	65
0	6	5	209	123
6	0	6	35	139
0	6	10	211	225
3	0	5	69	125
7	0	11	24	244

Using these data we setup an appended matrix containing the some of the elements in the equation below.

The appended matrix of the leftmost matrix would be the matrix Q. The appended matrix of the rightmost matrix would be the matrix d. What we want to find is second matrix in the equation which we denote as matrix a. We do this by applying the equation below.

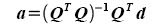
For my case I used excel to calculate and to construct the matrix but you could use other methods as well such as calculating it in scilab. I would not show the appended matrices but only the calculated matrix a.

Matrix a

-1.12E+01

16.47929

-3.72E-02

9.93E+01

-3.82E+00

-2.28E+00

1.88E+01

3.67E+01

-1.20E-02

-6.07E-03

-2.53E-03

1

Now we examine if these are correct. We use the equation

We use this to our previous data and try to see if we get the same [Yi, Zi] coordinates. The Table below shows the results and their difference from the known values.

Yr		Zr		Yi		Zi		delta Y		delta Z
99.26059921		36.6903502		100		36		0.739401		0.69035
89.53561712		71.7207949		90		72		0.464383		0.279205
89.92225298		110.57204		90		112		0.077747		1.42796
117.7563384		130.931302		117		131		0.756338		0.068698
153.9647225		167.560105		154		168		0.035278		0.439895
170.9055698		106.477688		171		106		0.09443		0.477688
152.1637482		69.0766455		152		69		0.163748		0.076645
206.1266372		43.4976498		205		44		1.126637		0.50235
191.0174924		185.15496		191		185		0.017492		0.15496
58.10852607		163.864721		57		164		1.108526		0.135279
46.48536116		99.8337782		46		100		0.485361		0.166222
57.41563756		42.3547116		58		42		0.584362		0.354712
80.32558877		208.104854		80		208		0.325589		0.104854
22.99597131		52.210583		23		52		0.004029		0.210583
35.14187397		180.953798		35		181		0.141874		0.046202
227.5075306		141.768338		228		142		0.492469		0.231662
173.3966707		226.951393		173		227		0.396671		0.048607
47.01195681		224.895651		47		225		0.011957		0.104349
136.650702		208.739956		137		208		0.349298		0.739956
188.2468657		65.2110463		188		65		0.246866		0.211046
208.1607909		123.1026		209		123		0.839209		0.1026
35.02876316		138.731433		35		139		0.028763		0.268567
210.7650967		225.019992		211		225		0.234903		0.019992
68.89331288		125.394012		69		125		0.106687		0.394012
23.20818217		244.182491		24		244		0.791818		0.182491
						Average		0.384953		0.297556

We see that there is little difference between the actual and the calculated values which means our calculated matrix a is approximately correct.

Now we use the calibration matrix a to predict the [Yi, Zi] coordinates of a known [Xw, Yw, Zw] coordinates then we compare it to the actual values. The results are below.

Xw	Yw	Zw			Yr		Zr		Yi		Zi		0.384953
7	0	10			23.18406822		222.368334		23		222		0.184068
0	0	11			101.6775298		250.622298		102		250		0.32247
0	8	0			242.8889262		19.3613035		242		19		0.888926
0	7	3			225.8096953		81.2166586		226		81		0.190305
0	6	7			209.1941664		163.542911		209		163		0.194166
0	7	11			230.3987701		244.881633		230		245		0.39877
											Average		0.363118

We see that our predictions correspond fairly well with our actual values. This further verifies that our calculated matrix a is correct. The image below is the grid and the blue dots are the points used to find the calibration matrix and the block dots are the points whose [Yi, Zi] coordinates were determined using the calibration matrix.

I give myself a grade of 10 for this activity since I have successfully calculated the calibration matrix and also verified its validity by predicting values for a set of real world coordinates.
Thank you for Jorge Presto and Billy Narag for collaborating with me in this activity.